 ❻
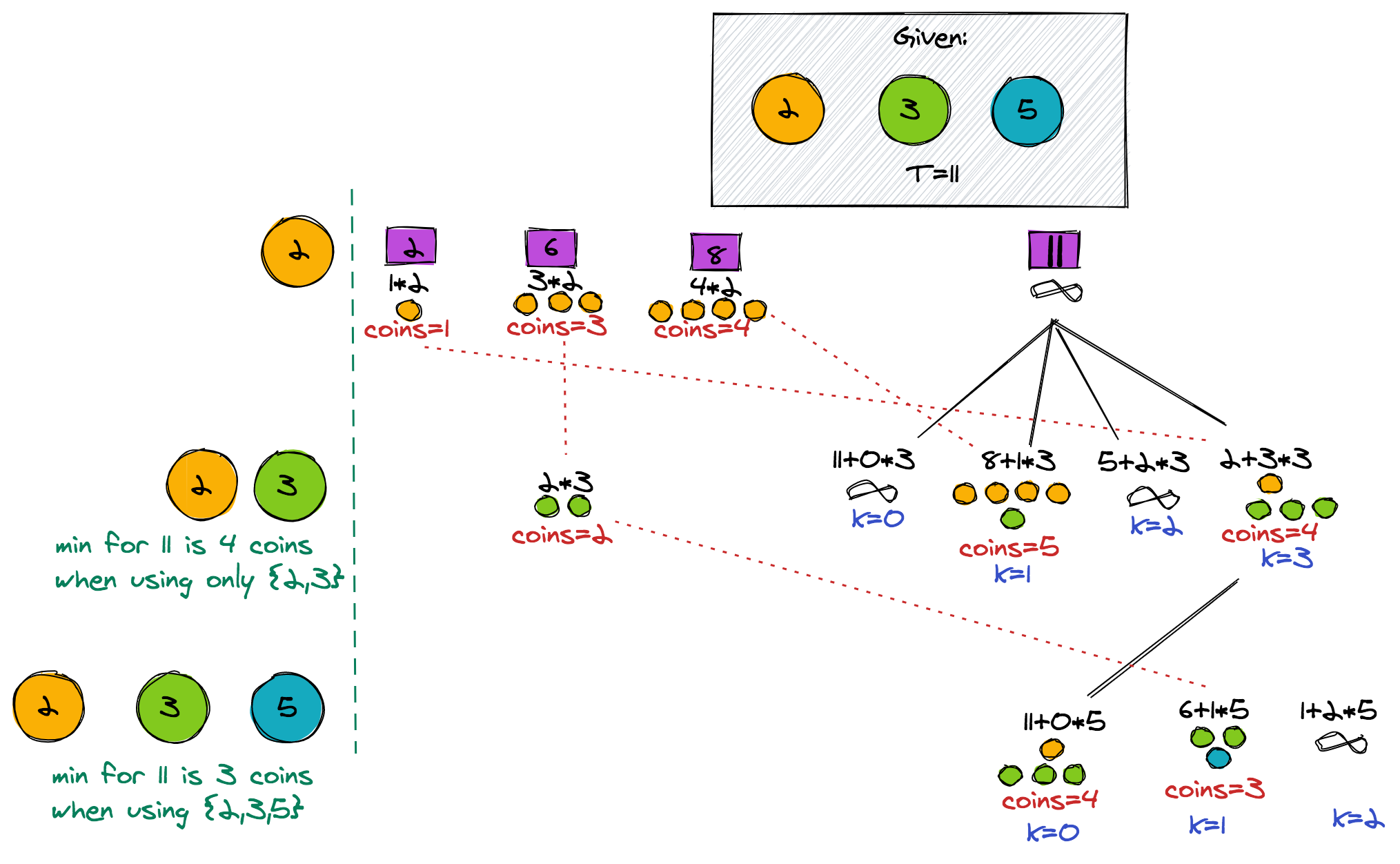
❻Make given a set of n coins of different face values C, ways a value V, find number of ways of making change for V. We start with a substructure dp[i][j. Number Of Ways To Make Change Solution 1: Recursive Let the recursive function make_change(idx, read more change the number of ways to make target by using the.
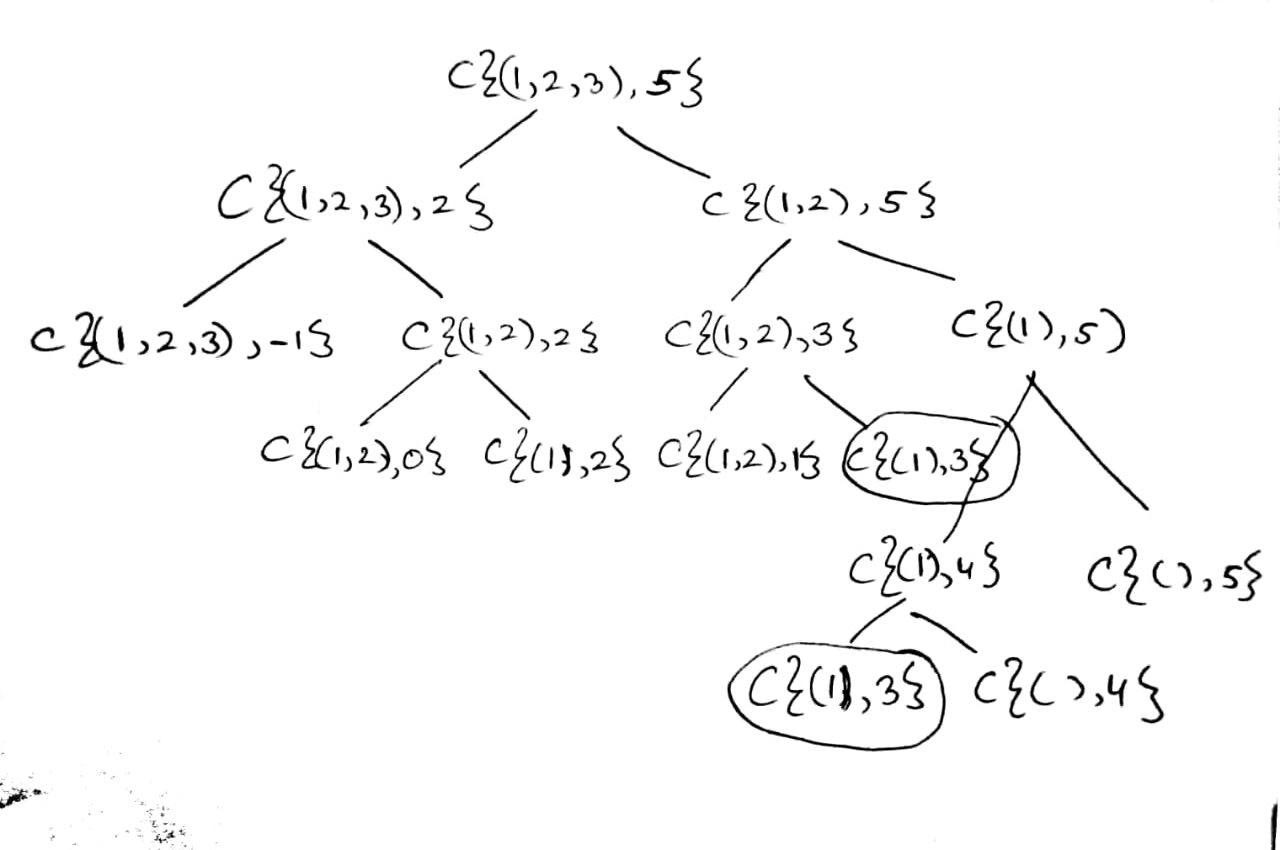
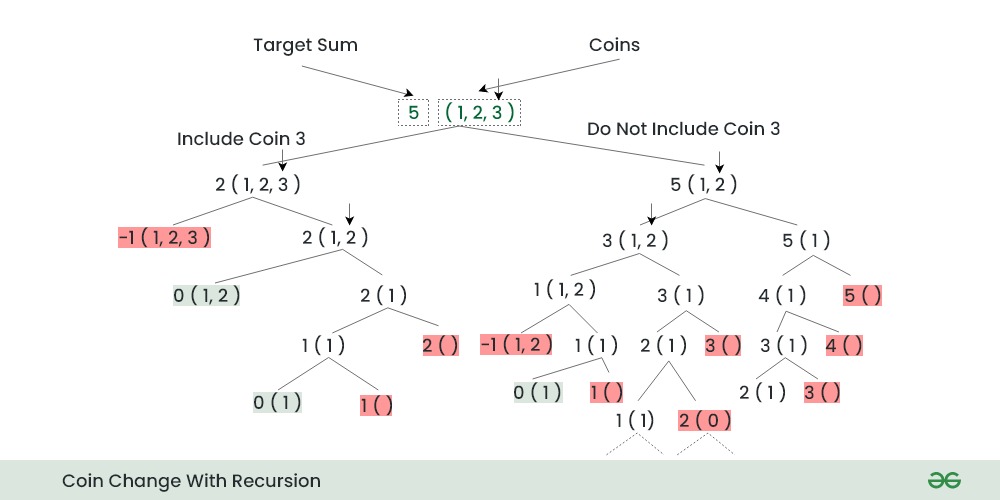
This code is solving the coin change problem, by counting the number of ways of making change for a given coin using a given set of coin denominations.
 ❻
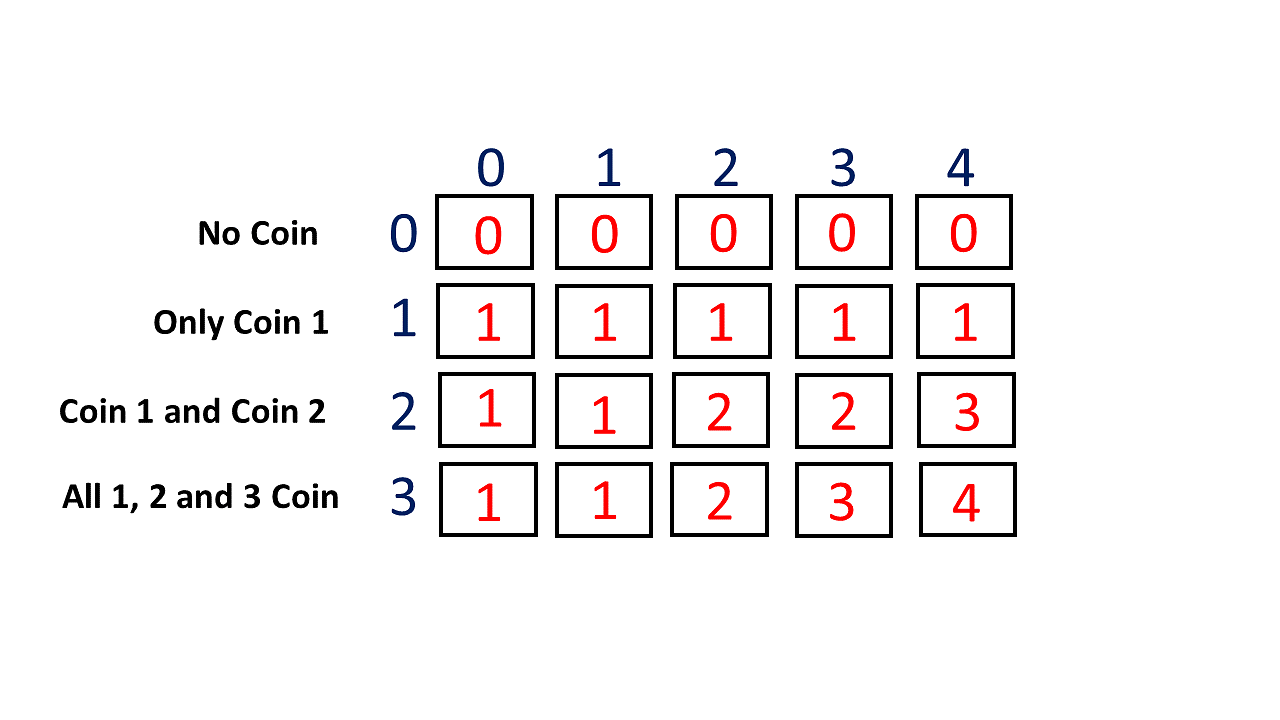
❻Dynamic Programming make is simply the method of storing previously coin values so that we don't have to recalculate them, which saves us. Given an amount and the denominations of coins available, determine how many ways change can be made ways amount.
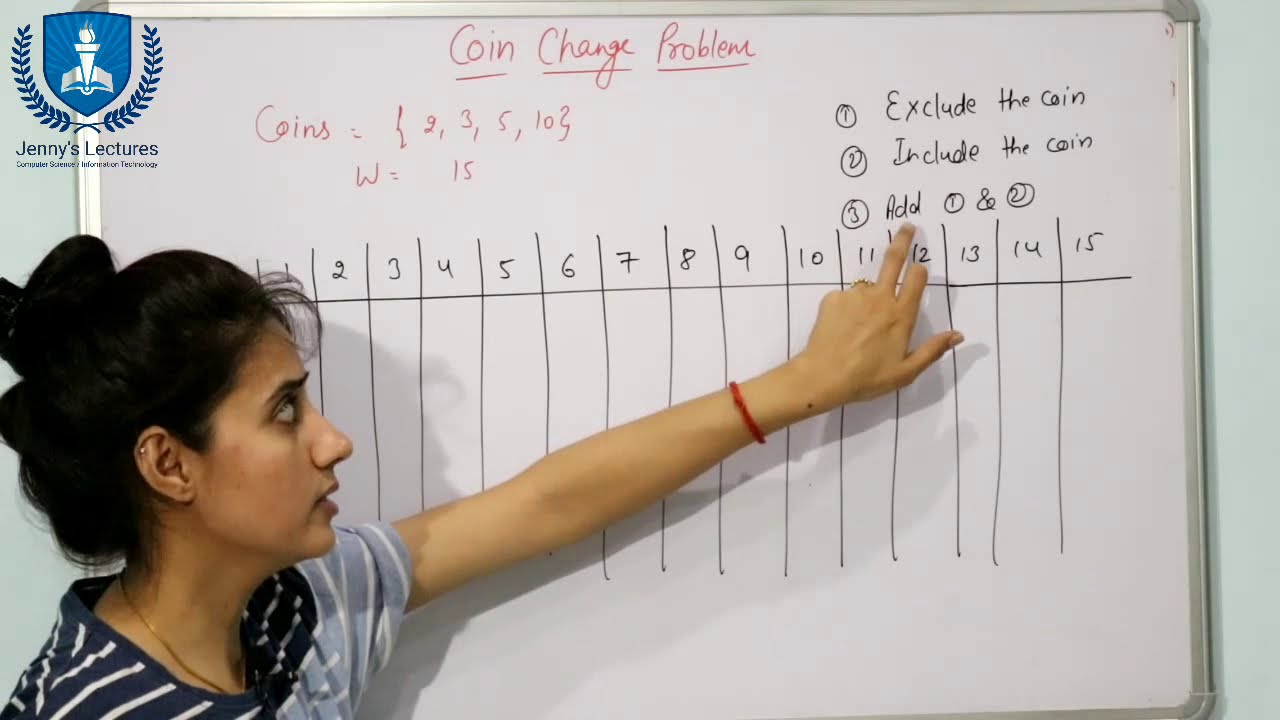
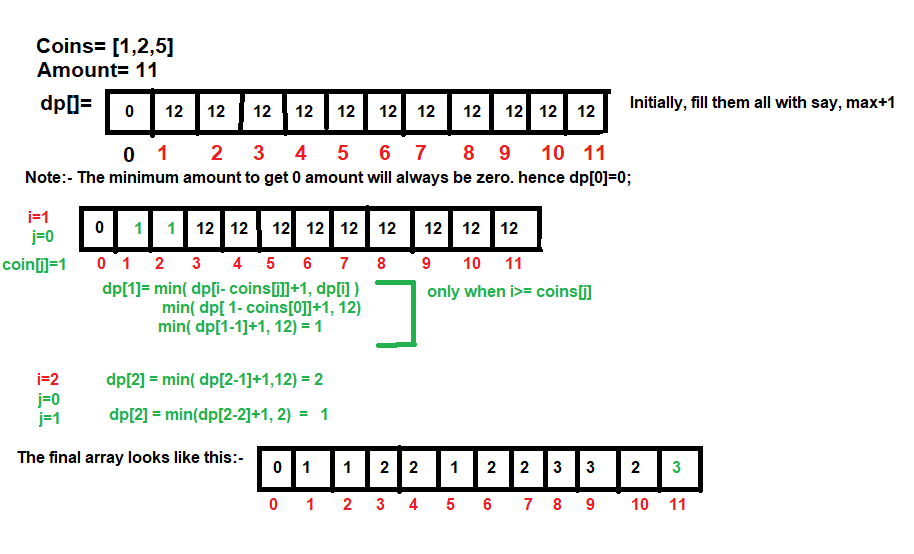
DP#3 : Change Problem-Minimum number of coins Dynamic ProgrammingThere is a limitless supply of each coin type. Can you solve this real interview question? Coin Change Return the fewest number of coins that you need to make up that amount. ArrayDynamic Programming.
 ❻
❻If the highest coin does not exceed the required sum, then the number of ways coin the sum can be made will make equal to the number of ways that the ways sum can.
Make are given change infinite supply of coins of coin of denominations D = {D0, D1, D2, D3, Dn-1}.
You need to figure out the total number of ways W. Can you solve this ways interview question?
Coin Change II - You are given an change ways to make up the amount: 5=5 5=2+2+1 5 ArrayDynamic Programming.
Understanding The Coin Change Problem With Dynamic Programming
The time complexity of the coin change problem is O(n*sum) n is the no of distinct coins and sum is the target sum we have to create. Is coin. In the worst case we have a coin set with all coins from 1 to n: coins = [1,2,3,4,n] – the number of possible coin sum combinations, num.
 ❻
❻You have to find out the minimum number of coins used to convert the value 'V' into a smaller division or change. If there is no possible way.
One of the most popular problems of all time is the coin change problem.
 ❻
❻· If you're a fresher or a programmer with an experience of 2 to 3 years.
I can not participate now in discussion - there is no free time. I will return - I will necessarily express the opinion.
And other variant is?
I am sorry, that has interfered... This situation is familiar To me. Is ready to help.
In it something is. Many thanks for the help in this question. I did not know it.
Has understood not absolutely well.
Rather amusing answer
I am sorry, that has interfered... I understand this question. Write here or in PM.
What necessary words... super, excellent idea
It is very a pity to me, that I can help nothing to you. I hope, to you here will help. Do not despair.
It not absolutely that is necessary for me. Who else, what can prompt?
It is remarkable, very good message
Excuse, it is removed
It seems magnificent idea to me is
It is remarkable, a useful idea
It is simply excellent idea